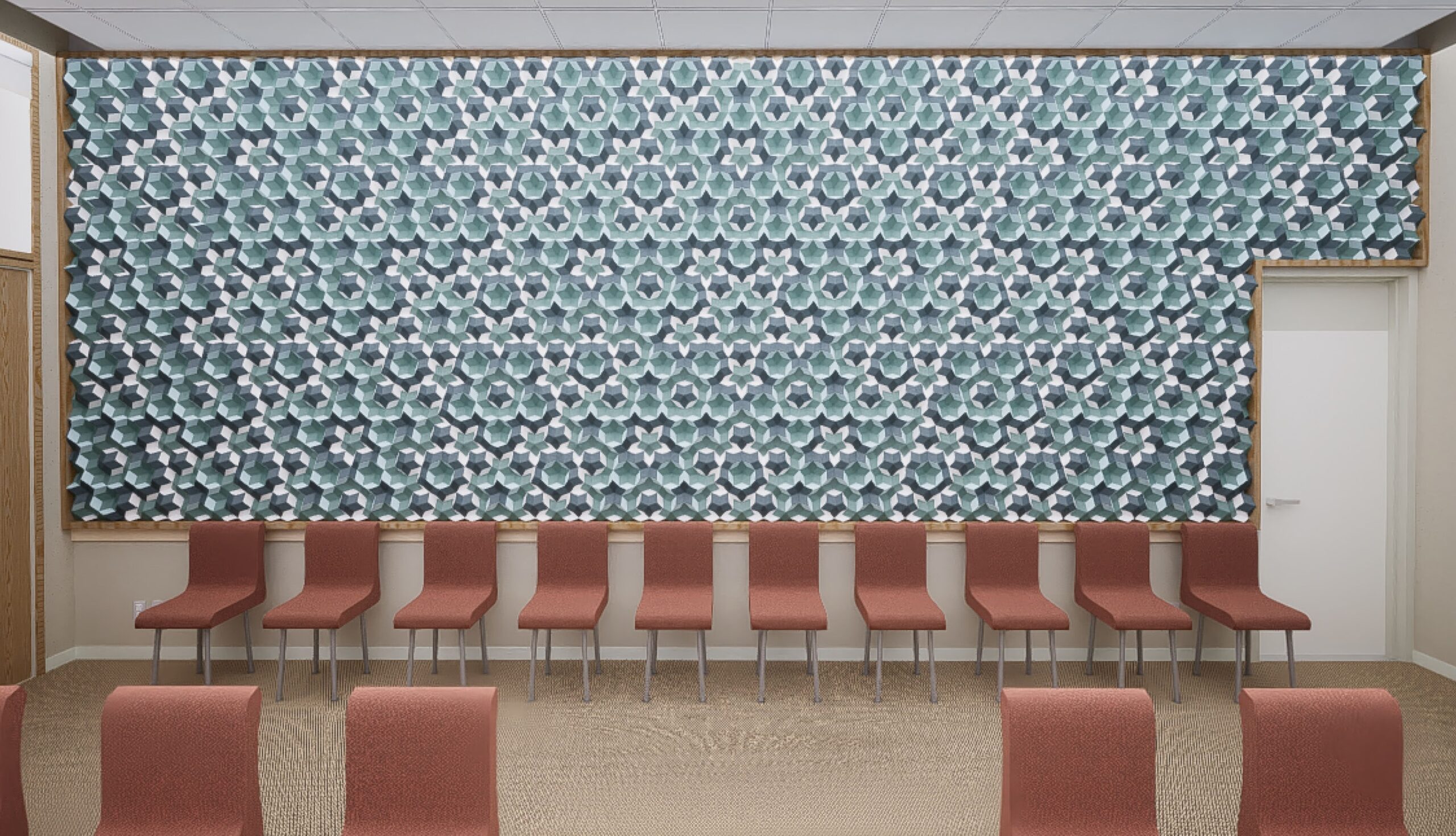
This study explores a convergence of order and disorder known as aperiodicity. It examines how Penrose tiling, showcasing local symmetries fading into disorder, has influenced design. Modern designers extend this concept into the 3D realm, particularly in acoustics, utilizing complex surfaces for controlled sound scattering. This research combines aperiodicity with a mathematical formula through parametric modeling. Simulations evaluate acoustic performance, informing a physical architectural installation. This work introduces a novel method to create 3D objects using parametric models, resulting in Wieringa Surfaces and Penrose Tiling. The study's significance lies in its innovative approach to sound scattering surfaces and aperiodic design derived from mathematical methods.